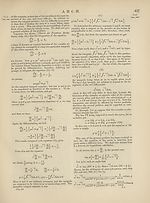Encyclopaedia Britannica > Volume 3, Anatomy-Astronomy
(424) Page 416
Download files
Complete book:
Individual page:
Thumbnail gallery: Grid view | List view

416
ARCH.
Arch.
By the nature of the centre of gravity,
u>: w' — CG : BG = CK : BL,
1 1 _ HG hg
BL : CK “ BL : CK :
HL GL
therefore w \ w' —
HG XXJLJ VX-
Now —— — —r = tan. <p — tan. ©
^ BL “ BL BL y
xxvx vxx, HK , t
and ^ = -7^? — qk = tan- 9 ~ tan- ? ?
HG _ GK
CK “ CK
hence it appears that
w vf — tan. <p — tan. <p': tan. <p' — tan. f".
If uf' denote the load on the next joint D, and <p'" the
angle which the line DE makes with the horizontal plane,
it will appear in the same way that
vf : w" = tan. f' — tan. <p": tan. <p" — tan. <p"',
and so on throughout the whole polygon: whence we
have this important proposition:
The vertical pressures on any two joints of the polygon,
whether adjoining or remote, are to one another as the dif¬
ferences of the tangents of the angles which the sides about
the joints make with the plane of the horizon.
52. From this proposition we may infer, that if p, p' de¬
note the angles which any two adjoining sides of the
polygon make with the horizontal plane, and w the load
on the joint at their intersection, then,
w — ^ (tan. p — tan. (A) ;
and in this formula, C denotes some constant quantity,
which is the same for all the angles of the polygon. This
is the general equation of the equilibrated polygon; and
it shows that the loads at the joints depend entirely on
the angles which the beams make with the horizontal
plane, and are independent of the lengths of the beams
themselves.
Equilibrated Arch.
53. We shall next investigate the differential equation
of an equilibrated arch, deducing it from the finite equa¬
tion of the equilibrated polygon.
Let us suppose an equilibrated polygon of a very great
number of sides (fig- lO)? and that a curve ABC passes
through all the joints: the sides of the polygon will then
be chords in the curve; and as the number of these may
be conceived to be greater than any assigned number, and
each shorter than any given line, they may be regarded
as elements of the curve, and as constituting it.
54. Let us suppose the curve ABC formed in this man¬
ner to be the intrados of an arch of a bridge, and that the
extrados is the line MDN, which may be curved or
straight. Let AC be the span, or greatest horizontal
width of the arch, and BH, which bisects AC at right
angles, its rise or height; also let BD be the thickness of
the arch of the crown : draw a straight line EDF perpen¬
dicular to DH, and draw AE, CF perpendicular to EF.
Let Vp be any indefinitely small part of the intrados
ABC. Draw PHQ perpendicular to the horizontal line
EF, meeting the extrados in R, and rp parallel to RP;
also draw PK perpendicular to DH, meeting rp in q, and
PT touching the intrados in p; and, referring the two
curves to the same axes DE, let us put
x = DQ the common abscissa,
y — PQ the ordinate of the intrados,
v — RQ the ordinate of the extrados,
p — angle TPK made by the tangent and horizontal linePK,
c’— DB the given value of y at the crown,
d'— AE the given value of y at either haunch.
Then, because Yp is a differential of the arc BP, we
have Yq = dx, and pq = dy.
55. The load on the arc BP is the gravitating matter
between it and DR, tne arc of tne extrados immediately ( Arch,
over it. This is expressed by the area BDRP; there-
fore the load on Yp, the differential of the arc, is (y — v)dx,
the differential of that area.
We have put f to denote the angle which a tangent at
P makes with any horizontal line ; similarly, let <p denote
the angle which a line touching the curve at p makes
with a horizontal line. If now the curve be considered
as a polygon of an infinite number of infinitely short sides,
and the lines which touch the curve at P and p as the pro¬
longations of two adjoining sides, then, by formula (A),
sect. 52, the vertical pressure at their intersection will be
C (tan. p — tan. <pj. But these infinitely short touching
lines may be regarded as forming Yp, the differential of
the curve, the load on which we have found to be
(y — v)dx; and, moreover, tan. <p — tan. p' = rf(tan. <p),
the differential of the trigonometrical tangent of p; there¬
fore, the relation between the intrados and extrados will
be expressed by this equation,
C c?(tan. <p) = (y — v)dx.
Now in all curves, tan. f and making dx constant,
(/(tan. <p) = let us, to make the members of the
equation homogeneous, put c2 instead of C, which is al¬
ways positive, and the above equation becomes
dx
= (y — v)dx ;
•(B).
and hence
d?y y _ v
dx? <? <?
This differential equation, which is of the second order,
and linear, or of the first degree, comprehends in it the
whole theory of the equilibrated arch. We may now de¬
duce from it the resolution of two problems.
Prob. 1. Having given the form of the intrados ABC,
to find that of the extrados.
Prob. 2. Having given the nature of the extrados, to
determine the intrados.
56. The first problem is easy, and may be resolved by
the differential calculus.
Ex. Let the intrados ABC be a segment of a circle
whose centre is O. Draw PO to the centre. Let angle
POB, and a = PO the radius of the circle ; then,
x — DO = PK = a sin. <p,
y — PQ — BD + BK = c'+a (1 — cos. <p),
dxzz. a cos. <p dtp, dy — a sin. <pd<p,
dv sin. © . _ d?y d<p
dx cos. p r dx cos/cp
cPy _ 1 _ sec.3 p
dx2 a cos.3 p a
Now, by formula (B),
y — v _ d?y _ sec.3 p
c2 dx? a
therefore y — v — sec.3 p.
But when * = 0, then v— y —c', p = 0, and sec. p = 1;
(?
therefore d — - and (? — ad ; hence we have
a
v — y — d sec.3 p.
This shows that the vertical line between the intrados
and extrados is always proportional to the cube of the
secant of the angle which the radius OP makes with the
perpendicular; a conclusion which agrees with section 25
of the preceding article.
57. The second problem, viz. having given the nature
ARCH.
Arch.
By the nature of the centre of gravity,
u>: w' — CG : BG = CK : BL,
1 1 _ HG hg
BL : CK “ BL : CK :
HL GL
therefore w \ w' —
HG XXJLJ VX-
Now —— — —r = tan. <p — tan. ©
^ BL “ BL BL y
xxvx vxx, HK , t
and ^ = -7^? — qk = tan- 9 ~ tan- ? ?
HG _ GK
CK “ CK
hence it appears that
w vf — tan. <p — tan. <p': tan. <p' — tan. f".
If uf' denote the load on the next joint D, and <p'" the
angle which the line DE makes with the horizontal plane,
it will appear in the same way that
vf : w" = tan. f' — tan. <p": tan. <p" — tan. <p"',
and so on throughout the whole polygon: whence we
have this important proposition:
The vertical pressures on any two joints of the polygon,
whether adjoining or remote, are to one another as the dif¬
ferences of the tangents of the angles which the sides about
the joints make with the plane of the horizon.
52. From this proposition we may infer, that if p, p' de¬
note the angles which any two adjoining sides of the
polygon make with the horizontal plane, and w the load
on the joint at their intersection, then,
w — ^ (tan. p — tan. (A) ;
and in this formula, C denotes some constant quantity,
which is the same for all the angles of the polygon. This
is the general equation of the equilibrated polygon; and
it shows that the loads at the joints depend entirely on
the angles which the beams make with the horizontal
plane, and are independent of the lengths of the beams
themselves.
Equilibrated Arch.
53. We shall next investigate the differential equation
of an equilibrated arch, deducing it from the finite equa¬
tion of the equilibrated polygon.
Let us suppose an equilibrated polygon of a very great
number of sides (fig- lO)? and that a curve ABC passes
through all the joints: the sides of the polygon will then
be chords in the curve; and as the number of these may
be conceived to be greater than any assigned number, and
each shorter than any given line, they may be regarded
as elements of the curve, and as constituting it.
54. Let us suppose the curve ABC formed in this man¬
ner to be the intrados of an arch of a bridge, and that the
extrados is the line MDN, which may be curved or
straight. Let AC be the span, or greatest horizontal
width of the arch, and BH, which bisects AC at right
angles, its rise or height; also let BD be the thickness of
the arch of the crown : draw a straight line EDF perpen¬
dicular to DH, and draw AE, CF perpendicular to EF.
Let Vp be any indefinitely small part of the intrados
ABC. Draw PHQ perpendicular to the horizontal line
EF, meeting the extrados in R, and rp parallel to RP;
also draw PK perpendicular to DH, meeting rp in q, and
PT touching the intrados in p; and, referring the two
curves to the same axes DE, let us put
x = DQ the common abscissa,
y — PQ the ordinate of the intrados,
v — RQ the ordinate of the extrados,
p — angle TPK made by the tangent and horizontal linePK,
c’— DB the given value of y at the crown,
d'— AE the given value of y at either haunch.
Then, because Yp is a differential of the arc BP, we
have Yq = dx, and pq = dy.
55. The load on the arc BP is the gravitating matter
between it and DR, tne arc of tne extrados immediately ( Arch,
over it. This is expressed by the area BDRP; there-
fore the load on Yp, the differential of the arc, is (y — v)dx,
the differential of that area.
We have put f to denote the angle which a tangent at
P makes with any horizontal line ; similarly, let <p denote
the angle which a line touching the curve at p makes
with a horizontal line. If now the curve be considered
as a polygon of an infinite number of infinitely short sides,
and the lines which touch the curve at P and p as the pro¬
longations of two adjoining sides, then, by formula (A),
sect. 52, the vertical pressure at their intersection will be
C (tan. p — tan. <pj. But these infinitely short touching
lines may be regarded as forming Yp, the differential of
the curve, the load on which we have found to be
(y — v)dx; and, moreover, tan. <p — tan. p' = rf(tan. <p),
the differential of the trigonometrical tangent of p; there¬
fore, the relation between the intrados and extrados will
be expressed by this equation,
C c?(tan. <p) = (y — v)dx.
Now in all curves, tan. f and making dx constant,
(/(tan. <p) = let us, to make the members of the
equation homogeneous, put c2 instead of C, which is al¬
ways positive, and the above equation becomes
dx
= (y — v)dx ;
•(B).
and hence
d?y y _ v
dx? <? <?
This differential equation, which is of the second order,
and linear, or of the first degree, comprehends in it the
whole theory of the equilibrated arch. We may now de¬
duce from it the resolution of two problems.
Prob. 1. Having given the form of the intrados ABC,
to find that of the extrados.
Prob. 2. Having given the nature of the extrados, to
determine the intrados.
56. The first problem is easy, and may be resolved by
the differential calculus.
Ex. Let the intrados ABC be a segment of a circle
whose centre is O. Draw PO to the centre. Let angle
POB, and a = PO the radius of the circle ; then,
x — DO = PK = a sin. <p,
y — PQ — BD + BK = c'+a (1 — cos. <p),
dxzz. a cos. <p dtp, dy — a sin. <pd<p,
dv sin. © . _ d?y d<p
dx cos. p r dx cos/cp
cPy _ 1 _ sec.3 p
dx2 a cos.3 p a
Now, by formula (B),
y — v _ d?y _ sec.3 p
c2 dx? a
therefore y — v — sec.3 p.
But when * = 0, then v— y —c', p = 0, and sec. p = 1;
(?
therefore d — - and (? — ad ; hence we have
a
v — y — d sec.3 p.
This shows that the vertical line between the intrados
and extrados is always proportional to the cube of the
secant of the angle which the radius OP makes with the
perpendicular; a conclusion which agrees with section 25
of the preceding article.
57. The second problem, viz. having given the nature
Set display mode to:
![]() Universal Viewer |
Universal Viewer | ![]() Mirador |
Large image | Transcription
Mirador |
Large image | Transcription
Images and transcriptions on this page, including medium image downloads, may be used under the Creative Commons Attribution 4.0 International Licence unless otherwise stated. ![]()
| Encyclopaedia Britannica > Encyclopaedia Britannica > Volume 3, Anatomy-Astronomy > (424) Page 416 |
|---|
| Permanent URL | https://digital.nls.uk/193762860 |
|---|
| Attribution and copyright: |
|
|---|---|
| Shelfmark | EB.16 |
|---|---|
| Description | Ten editions of 'Encyclopaedia Britannica', issued from 1768-1903, in 231 volumes. Originally issued in 100 weekly parts (3 volumes) between 1768 and 1771 by publishers: Colin Macfarquhar and Andrew Bell (Edinburgh); editor: William Smellie: engraver: Andrew Bell. Expanded editions in the 19th century featured more volumes and contributions from leading experts in their fields. Managed and published in Edinburgh up to the 9th edition (25 volumes, from 1875-1889); the 10th edition (1902-1903) re-issued the 9th edition, with 11 supplementary volumes. |
|---|---|
| Additional NLS resources: |
|